Overview

Electric devices and their individual component parts have eigenmodes that are determined by their mechanical properties and their geometry. Vibration increases when the excitation force resonates with the electromagnetic device’s eigenmode. Also, electric devices and their component parts deform when a load is applied to them. Even in a simple form like a cantilever beam, the displacement direction differs depending on the direction of the load, its position, and the frequency. For this reason, it is necessary to evaluate the eigenmode and displacement for an electric device and its component parts in order to understand these phenomena.
In this Application Note, the displacement is obtained for three simple cantilever beam models with different mesh conditions when their eigenmode or a concentrated load is applied to them, and the displacement is then compared with theoretical values.
Eigenmode
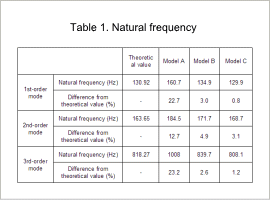
The first-order eigenmodes for Models A (coarse mesh, first-order elements ), B (fine mesh, first-order elements ), and C (coarse mesh, second-order elements ) are shown in fig. 1 and their first- through third-order natural frequencies are shown in table 1. These show that the deformation direction of the first-order eigenmode can be found even with a coarse mesh, but in order to keep the natural frequency including bending within around 5% off from the theoretical value, it is necessary to either use a finer mesh or use second-order elements.

Displacement
The Z-direction displacements for Models A, B, and C are shown in fig. 2, and their maximum Z-direction displacements are shown in table 2. These show that the deformation can be found approximately with a coarse mesh, but in order to keep the maximum displacement including bending within around 5% off from the theoretical value, it is necessary to either use a finer mesh or use second-order elements.




