Fig. 1

On the telephone recently my 8-year-old grandson asked if I knew the equation \(E = mc^2\). When I said I’d heard of it, he proceeded to explain: \(E\) is energy, \(m\) is mass, and \(c\) is the speed of light.

This reminded me of a crowd of students waiting to enter the examination hall for their final examinations in electrical engineering. One of them stood by the door, and in a loud voice announced \( “V = IR!” \) — a gift to his fellow-students, and perhaps the last words of advice before starting a career as an electrical engineer.

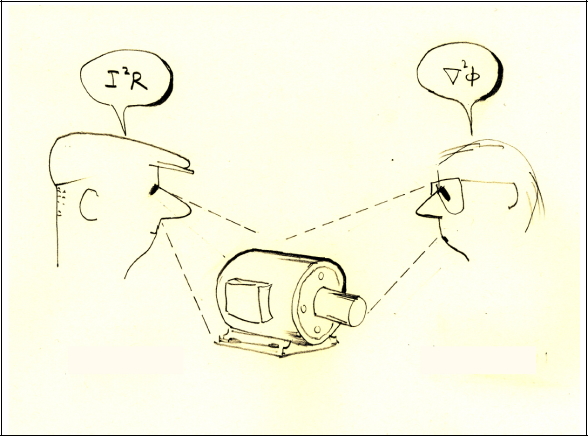
These equations are what we might call notional equations. We have hundreds of them in engineering. As they stand, they are often not precise. The units are often not stated, yet without them the equations are useless. Often there are coefficients which make the equation more precise : for example, in the classical voltage equation for an AC machine we see the winding factor, which isolates the fundamental time-harmonic of the generated voltage. But the notional form of this equation is just \( “V = dψ/dt” \) or \( “V = jωψ” \) or “Volts = flux-linkage × frequency”. Take another example, \(B = μH\). Flux-density equals permeability times magnetizing force — but in what units?

Notional equations are a way of expressing fundamental laws and principles in ordinary speech. In describing the operation of a mechanism or system, engineers often use them. Another example is “Torque × Speed = Volts × Amps”. That formula is almost never a precise statement, because it lacks the coefficients mentioned earlier, and it ignores nonlinearities and parasitic effects like friction, as well as second-order effects like temperature variations. But in its general sense it applies to almost all electric machines. What these machines do is to convert energy between electrical and mechanical forms, and this conversion usually takes place with minimal losses, so the equation describes a process of lossless power conversion. Of course we know there is no such thing as lossless power conversion; but we also know that machines are designed to be highly efficient, so the equation is nearly true. The notional equation describes the essence of the conversion process. Power in equals power out. Or very nearly so.

This particular equation derives from two other notional equations, “Volts = Flux × Speed” and “Torque = Flux × Current”. Generally speaking, if you want a given motor to rotate faster, you need to supply more voltage; or failing that, reduce the flux. Reducing the flux will reduce the torque per ampere, by the second notional equation. If we’re lucky we might find that the product Volts × Amps remains the same, and so does the Torque × Speed. The flux goes down. The volts stay the same. The speed goes up. The amps stay the same but the torque goes down. This describes the principle of flux-weakening to achieve constant-power operation. In this form, using notional equations, it applies equally to the DC machine and the AC machine — indeed, to almost any machine. But it tells us nothing about the details. It doesn’t mention that in the DC machine the process involves a reduction of field current, while in the AC machine it involves changes in the operation of a pulse-width-modulated inverter to reduce the magnetizing component of the stator current while sustaining the torque-producing component. The details are complicated! But the simple essence remains the same, expressed by the notional equations.

So while the notional equations leave out all the engineering detail, nevertheless they express profound natural laws and principles.

Another example from our daily life in analytical engineering is Laplace’s equation, which in its simplest scalar form is \( \nabla ^2 \phi = 0\). We can say that a finite-element program solves Laplace’s equation. That sounds simple, because we’re quoting Laplace’s equation as a notional equation. But if we look into the details, we may find that the finite-element program is “solving Laplace’s equation” simultaneously in 10,000,000 elements with nonlinear properties that are extremely different in different regions or groups of elements, and in some of the elements boundary conditions are applied to constrain the solution process. When we describe it like that, the process sounds extremely complex (which of course it is), yet the expression \( \nabla ^2 \phi = 0\) sounds terribly simple.

We know that the solution of Laplace’s equation can be expressed in terms of flux-lines and equipotentials; and even with complex geometry we know that in linear regions at least, the flux-lines and the equipotentials will be orthogonal, forming curvilinear squares. We can make this sound more technical by quoting the Cauchy-Riemann conditions — using this mighty cornerstone of field theory as a notional equation in casual conversations with other engineers, and even using the principle of orthogonality to check by visual inspection that the finite-element solutions appear to be correct.

A notional equation often captures a process of fantastic complexity in a simple statement. Years ago I would often see an old colleague every year at a well known engineering conference, and we made a point of having a walk together, as a reminder of happy early days when we used to walk to work together. He was a specialist in motor control, and I asked him “What is the essential idea in field-oriented control?” — for, to be sure, it seemed very complicated to me and I really had not grasped the basis of it. Straightaway he answered, “Torque control. Torque equals flux times current. Just as in a DC machine. Control an AC machine like a DC machine”.

Wouldn’t it be marvellous if everything the professors tell their students was as clear and simple as that? My friend was not a professor. Just a plain engineer. But he had a firm grasp on the principles of his subject, and how eloquently he used a notional equation to answer an innocent question from a non-specialist.

I will finish with one more example from a training seminar. We were discussing power factor. An engineer put up his hand and said, “I don’t care about power factor, because all my motors are inverter-fed”. Another engineer put up his hand and said, “Oh yes you do, because one-over-the-power-factor (1/PF) is the volt-amperes per watt, and that’s the size of inverter you need for a motor of given power”. Here of course the notional equation is PF = Watts/(Volts × Amps), but the key idea in this story was not PF itself but 1/PF. That’s not so much a notional equation, but more of a notional operation. Taking the reciprocal is surely one of the commonest notional operations in engineering. We turn things upside-down to see them from a different point of view.

As an exercise, why not write down a few notional equations from your experience, and explain them in words. See how many of them involve complex physical processes like pulse-width modulation in inverters, and see how many of them involve mathematical operations like addition, subtraction, multiplication, division, differentiation, integration, — or the solution of large numbers of nonlinear simultaneous equations! Take a notion like power factor, or permeability, or inductance, or resistance, or velocity, and ask yourself what is the meaning of its reciprocal. Take two notions, such as voltage and current, and ask yourself what is the meaning of their product, or their quotient. You don’t need to derive any equations, but simply interpret the meaning. Go through your conversations at the end of the day, and try to count how many notional equations you used in your work or in conversations!